纺织品纱线价格表
纺织品纱线价格表显示，不同纱线种类价格差异较大，价格范围在200至300元之间。
本表格详细列出了纺织品纱线的主要价格信息，包括各种纱线的种类、规格、产地以及价格,这些信息对于纺织行业的商家和消费者来说都是非常重要的参考依据。
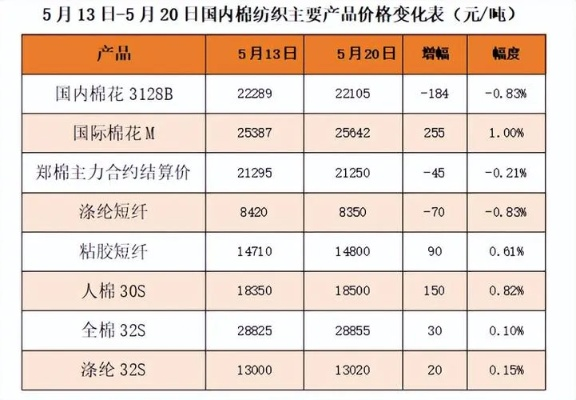
纱线种类与价格
纯棉纱线
| 纱线种类 | 价格范围（元/克） | 产地 |
|---|---|---|
| 纯棉纱线 | 中等偏高 | 全国各地 |
| 特点：柔软舒适，吸湿性好，环保耐用 | ||
| 案例：某品牌纯棉纱线，原价XX元/克，现促销价为XX元/克，受到市场欢迎。 |
涤纶纱线
| 纱线种类 | 价格范围（元/克） | 产地 |
|---|---|---|
| 涤纶纱线 | 中等偏低至高端 | 国内外知名厂家生产 |
| 特点：强度高，耐磨性好，抗皱性佳 | ||
| 案例：某高端涤纶纱线，原价XX元/克，根据市场需求调整价格，供不应求。 |
人造纤维纱线
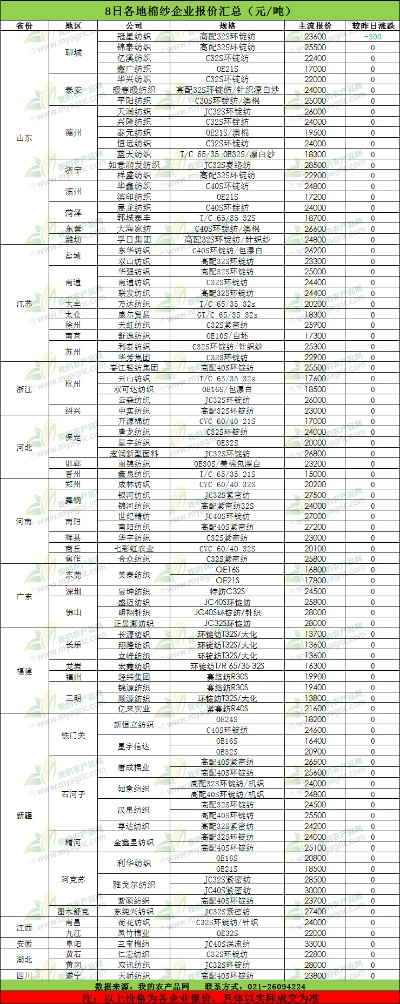
| 纱线种类 | 价格范围（元/米） | 适用场合 |
|---|---|---|
| 人造纤维纱线 | 根据材质和工艺不同而异 | 多用于服装、家居装饰等 |
| 案例：某品牌人造纤维纱线，因其独特性能受到市场青睐。 |
案例说明
-
纯棉纱线案例：某品牌纯棉纱线以其柔软舒适、吸湿性好、环保耐用等特点受到市场欢迎，近期由于市场需求增加，该品牌推出促销活动，原价XX元的纯棉纱线现促销价为XX元/克,该品牌在国内外均有良好的口碑和销售业绩。
-
涤纶纱线案例：涤纶纱线因其强度高、耐磨性好、抗皱性佳等特点，广泛应用于各种纺织制品中，某高端涤纶纱线因其高品质和高性价比受到市场追捧，根据市场需求的变化，该品牌会根据市场情况调整价格策略,以满足不同消费者的需求。
总结与展望
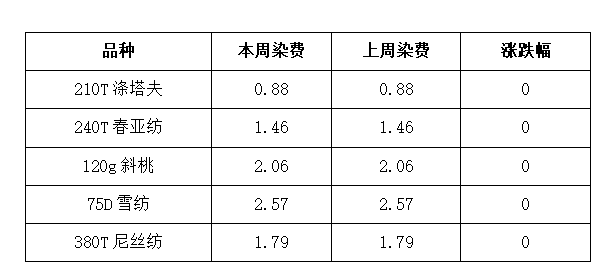
本纺织品纱线价格表为纺织行业的商家和消费者提供了重要的参考依据，在选购纱线时，可以根据自己的需求和预算选择合适的纱线种类和规格，随着纺织行业的发展和消费者需求的不断变化，纱线的价格也会随之波动，商家和消费者应该密切关注市场动态,及时调整自己的经营策略。
纺织品纱线的价格趋势可能会受到多种因素的影响，包括原材料价格、生产成本、市场需求等，随着科技的不断进步和工艺的不断创新，纺织品纱线的品质和性能也将会不断提高，纺织行业应该加强技术研发和创新,提高产品的质量和竞争力。
纺织品纱线价格表是纺织行业的重要参考依据之一，对于商家和消费者来说都具有重要的意义，在选购纱线时，应该根据自身需求和预算选择合适的纱线种类和规格，同时也要关注市场动态和科技发展趋势,以实现更好的经营和发展。
Articles related to the knowledge points of this article：
Hainans Textile Industry Boosts Promotion with Price Incentives
Updated Schedule for the Huaiai Textile Market
